Slope Calculator – Find the Slope of a Line

Use the slope calculator to find the slope of a line that passes through 2 points or solve a coordinate given m. Plus, the calculator will also solve the slope-intercept form of a line.

Calculate Using the Following:

  • 2 points
  • 1 point, slope, & distance
  • 1 point, slope, and x or y

Find the Slope Given 2 Points

Find a Point Given 1 Point, Slope, and Distance

Find a Point Given 1 Point, Slope, and an x or y Value

Slope, Angle, & Distance:

Slope, Angle, & Distance

The slope of the line connecting (1, 3) and (2, 5) is 2

Slope (m): 2
Angle (θ): 63.4349°
Distance: 2.2361
Δx: 1
Δy: 2

Slope Intercept Form:
(y = mx + b)
y = 2x + 1

Steps to Find Slope

Start with the slope formula

m = (y2 - y1) (x2 - x1)

Substitute point values in the formula

m = (5 - 3) (2 - 1)

Simplify each side of the equation

m = (5 - 3) (2 - 1) = 2 1

Solve for slope (m)

m = 2

How to Find the Slope of a Line Given 2 Points

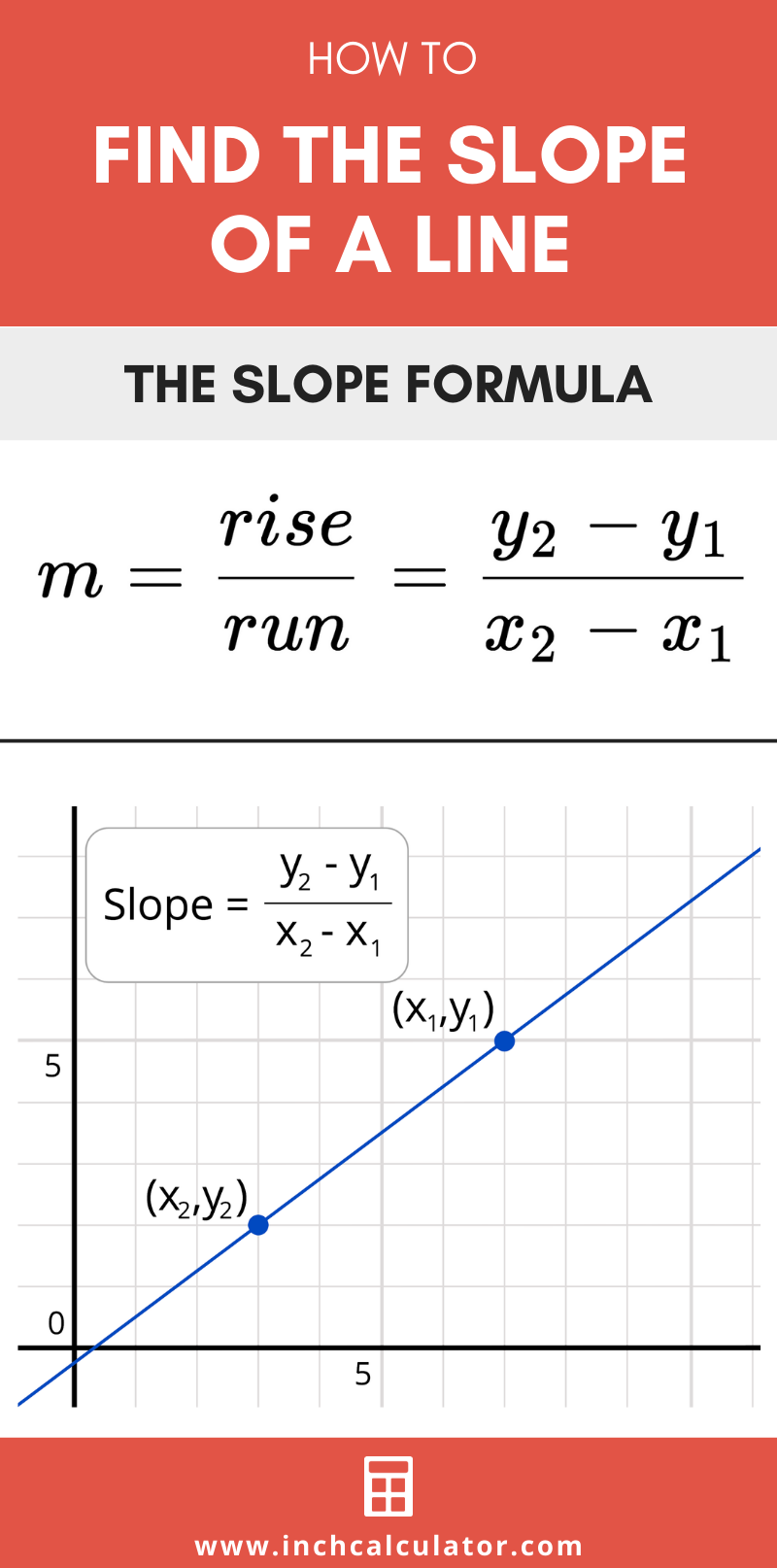
Slope is the angle of a line on a graph. It can be found by comparing any 2 points on the line. A point is an x and y value of a cartesian coordinate on a grid.

Slope m is equal to the rise between two coordinates on a line over the run. Rise is the vertical increase of the line, and run is the horizontal increase.

The Slope Formula

Slope, represented as m, can be found using the following formula:

slope = y2 – y1 x2 – x1

Thus, the slope m is equal to y2 minus y1 , divided by x2 minus x1 .

the slope formula showing m is equal to rise over run, or y2 minus y1 divided by x2 minus x1

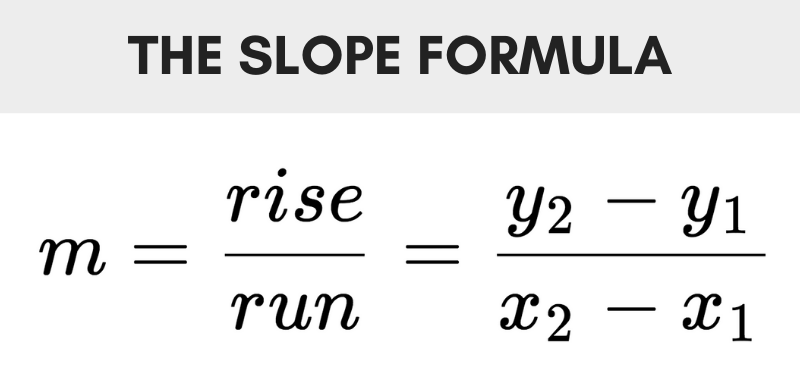
Use the Formula to Find Slope

To find the slope of a line, start by finding 2 points along a line and find their x and y values. The value of x is the horizontal distance of the point from the vertical y-axis, and y is the vertical distance of the point from the horizontal x-axis.

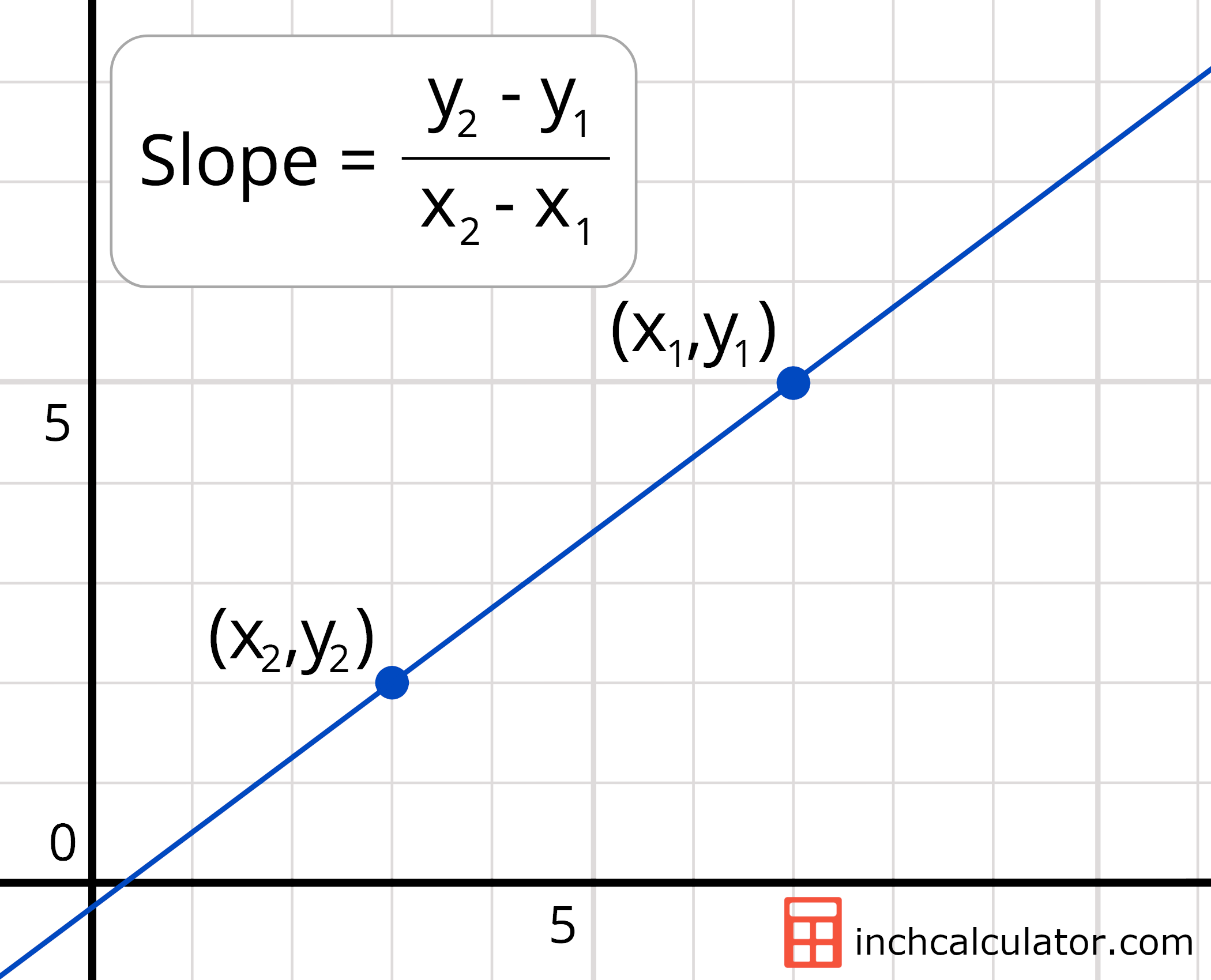
Graph showing a line and the slope formula to solve for m

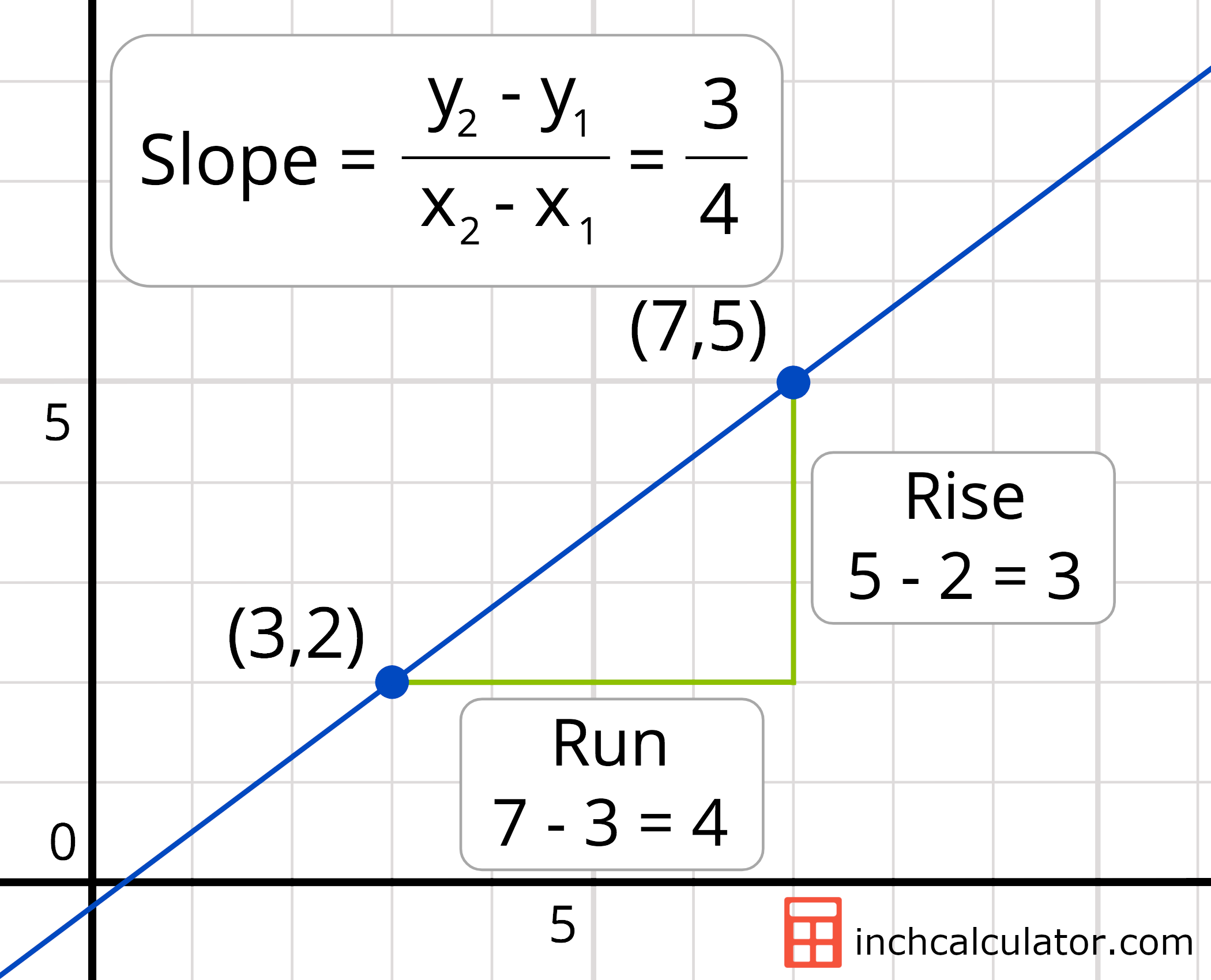
For example, let's find the slope of a line that passes through the points (3,2) and (7,5).

Start with the slope formula:

m = (y2 – y1) (x2 – x1)

Replace the x and y values with the coordinate's x and y values, then solve.

m = 5 – 2 7 – 3
m = 3 4

Thus, the slope m is equal to 3 4

graph showing how to solve the slope of a line passing through coordinates (3,2) and (7,5)

How to Find Slope-Intercept Form

A linear line can be expressed using slope-intercept form, which is an equation representing the line. Slope-intercept form can be solved using m and one point on the line.

Slope-Intercept Form Equation

The equation of a linear line can be expressed using the following equation, where m is the slope of the line, and b is the y-intercept value.

y = mx + b

Thus, the equation representing a line using slope-intercept form is the y value of a coordinate on the line is equal to the x value of the coordinate times m, plus the y-intercept b.

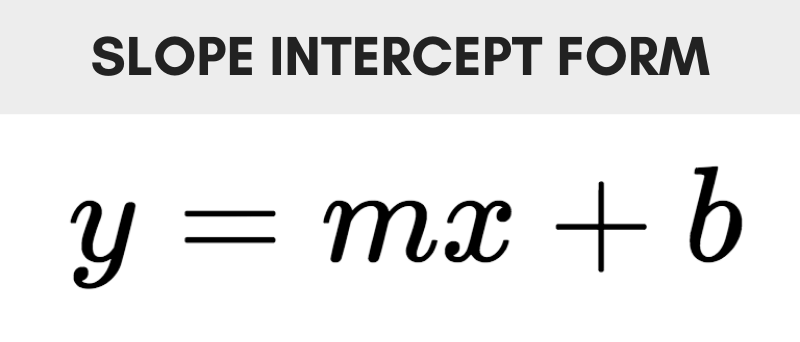
The slope-intercept form equation showing that y equals mx plus b

For example, let's solve for b, given a slope of 1/2 and a point (5,4).

Substitute for m:

y = 1 2 × x + b

Substitute the point values for x and y:

4 = 1 2 × 5 + b

Solve for b:

4 = 2.5 + b
4 – 2.5 = b
1.5 = b

Substitute b in the equation to find the slope-intercept form:

y = 1 2 x + 1.5

How to Find a Point Given 1 Point, Slope, and Distance

Points on a line can be solved given the slope of the line and the distance from another point. The formulas to find x and y of the point to the right of the point are:

x2 = x1 + d (1 + m2)

y2 = y1 + m × d (1 + m2)

The formulas to find x and y of the point to the left of the point are:

x2 = x1 + -d (1 + m2)

y2 = y1 + m × -d (1 + m2)

How to Convert Slope to Angle

The angle of a line in degrees can be found from the inverse tangent of the slope m.

θ = tan-1(m)

For example, if m = 5, then the angle in degrees is tan-1(5).

Our rise and run to degrees converter can help calculate the value in degrees given the rise and run of a line.

How to Convert Angle to Slope

It's also possible to convert an angle in degrees to slop, which is equal to the tangent of the angle.

m = tan(θ)

For example, if angle = 72, then m is equal to tan(72).

How to Find the Distance Between 2 Points

The formula to find the distance d between two points on a line is:

d = √((x2 – x1)2 + (y2 – y1)2)

How to Solve the Delta of x and y

The delta of x and y, expressed using the symbol Δ, is simply the absolute value of the distance between the x values or y values of two points.

The delta of x can be solved using the formula:

Δx = x2 – x1

The delta of y can be solved using the formula:

Δy = y2 – y1

Infographic showing how to find the slope of a line using the slope formula, which states that slope is equal to rise over run